Trig Functions - Graphing Cheat Sheet.UPDATED with different formula versions. Text books make graphing trig functions so complicated. I have simplified the process so that even below average students can follow the process easily and graph trig functions without frustration. See full list on brighthubeducation.com.
Score higher
Learning anything is easy
Learning on the go
Shop for books and more
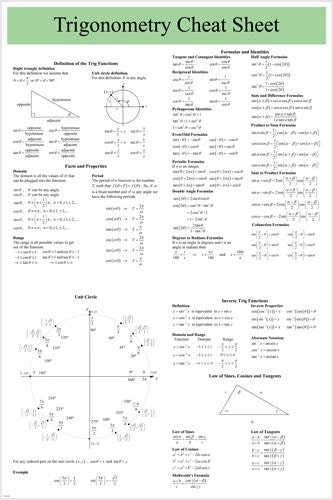
Trigonometry For Dummies From Trigonometry For Dummies, 2nd Edition by Mary Jane Sterling Trigonometry is the study of triangles, which contain angles, of course. Get to know some special rules for angles and various other important functions, definitions, and translations. Sines and cosines are two trig functions that factor heavily into any study of trigonometry; they have their own formulas and rules that you’ll want to understand if you plan to study trig for very long.
Formulas to Help You in Trigonometry Many of the formulas used in trigonometry are also found in algebra and analytic geometry. But trigonometry also has some special formulas usually found just in those discussions. A formula provides you a rule or equation that you can count on to work, every single time. A formula gives a relationship between particular quantities and units. The main trick to using formulas is to know what the different letters represent. In the formulas given here, you have: r (radius); d (diameter or distance); b (base or measure of a side); h (height); a, b, c (measures of sides); x, y (coordinates on a graph); m (slope); M (midpoint); h, k (horizontal and vertical distances from the center); θ (angle theta); and s (arc length). The formulas particular to trigonometry have: sin (sine), cos (cosine), and tan (tangent), although only sin is represented here.
Special Right Triangles Every right triangle has the property that the sum of the squares of the two legs is equal to the square of the hypotenuse (the longest side). The Pythagorean
theorem is written: a2 + b2 = c2. What’s so special about the two right triangles shown here is that you have an even more special relationship between the measures of the sides — one that goes beyond (but still works with) the Pythagorean theorem. When you have a 306090 right triangle, the measure of the hypotenuse is always twice the measure of the shortest side, and the other leg is always
or about 1.7 times as big as the shortest side. With the isosceles right triangle, the two legs measure the same, and the hypotenuse is always
or about 1.4 times as long as those two legs.
Right Triangle Definitions for Trigonometry Functions The basic trig functions can be defined with ratios created by dividing the lengths of the sides of a right triangle in a specific order. The label hypotenuse always remains the same — it’s the longest side. But the designations of opposite and adjacent can change — depending on which angle you’re referring to at the time. The opposite side is always that side that doesn’t help make up the angle, and the adjacent side is always one of the sides of the angle.
Coordinate Definitions for Trigonometry Functions The trig functions can be defined using the measures of the sides of a right triangle. But they also have very useful definitions using the coordinates of points on a graph. First, let let the vertex of an angle be at the origin — the point (0,0) — and let the initial side of that angle lie along the positive xaxis and the terminal side be a rotation in a counterclockwise motion. Then, when the point (x,y) lies on a circle that’s intersected by that terminal side, the trig functions are defined with the following ratios, where r is the radius of the circle.
Signs of Trigonometry Functions in Quadrants An angle is in standard position when its vertex is at the origin, its initial side is on the positive xaxis, and the terminal side rotates counterclockwise from the initial side. The position of the terminal side determines the sign of the various trig functions of that angle. The following shows you which functions are positive — and you can assume that the other functions are negative in that quadrant.
Degree/Radian Equivalences for Selected Angles As you study trigonometry, you'll find occasions when you need to change degrees to radians, or vice versa. A formula for changing from degrees to radians or radians to degrees is:
The formula works for any angle, but the most commonly used angles and their equivalences are shown below.
Laws of Sines and Cosines The laws of sines and cosines give you relationships between the lengths of the sides and the trig functions of the angles. These laws are used when you don’t have a right triangle — they work in any triangle. You determine which law to use based on what information you have. In general, the side a lies opposite angle A, the side b is opposite angle B, and side c is opposite angle C.
Exact Trigonometry Functions for Selected Acute Angles Using the lengths of the sides of the two special right triangles — the 306090 right triangle and the 454590 right triangle — the following exact values for trig functions are found. Using these values in conjunction with reference angles and signs of the functions in the different quadrants, you can determine the exact values of the multiples of these angles.
Dummies Recommends
From Around the Web
Trig Identities for PreCalculus How to Solve for a Missing Right Triangle Length Understanding Trigonometry Terms How to Use SohCahToa to Find the Trig Functions of a Right Triangle How to Graph Sine, Cosine, and Tangent Copyright © 2014 & Trademark by John Wiley & Sons, Inc. All rights reserved.

Here is list of cheat sheets and tables that I've written. All of the cheat sheets come in two version. A full sized version and a 'reduced' version. The reduced version contains all the information that the full sized version does except that each page from the full sized version has been reduced so that each page of the reduced version is two pages from the full version. With the exception of the Complete Calculus Cheat Sheet and the Integrals Cheat Sheet all the reduced versions will fit on one piece of paper. All of these are pdf files and so you will need the Adobe Viewer to view them. You can download the latest version here.
If you wish to link to any of these files the URL for each one of them is also given.
Algebra Cheat Sheet - This is as many common algebra facts, properties, formulas, and functions that I could think of. There is also a page of common algebra errors included. Currently the full version of the cheat sheet is 4 pages long.Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,31,N
Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,33,N
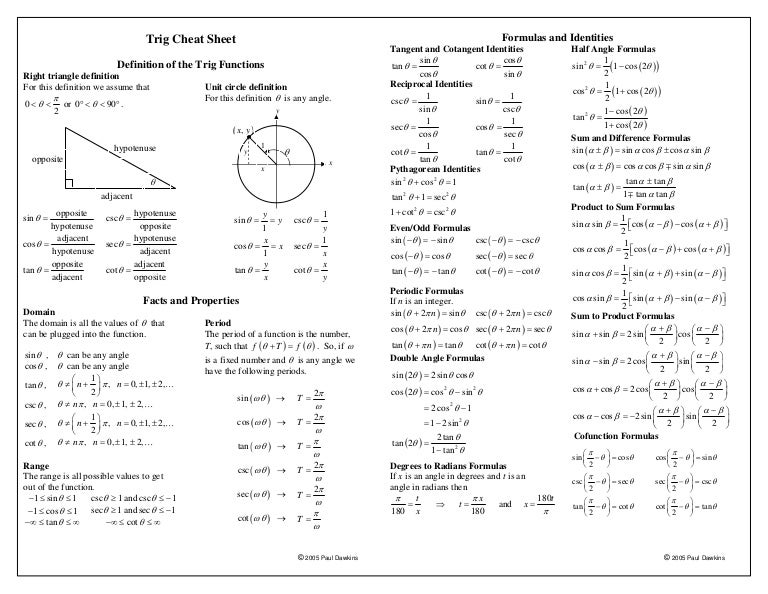 Complete Calculus Cheat Sheet - This contains common facts, definitions, properties of limits, derivatives and integrals. Most of the information here is generally taught in a Calculus I course although there is some information that is generally taught in a Calculus II course included as well. The full sized version is 11 pages.
Complete Calculus Cheat Sheet - This contains common facts, definitions, properties of limits, derivatives and integrals. Most of the information here is generally taught in a Calculus I course although there is some information that is generally taught in a Calculus II course included as well. The full sized version is 11 pages. 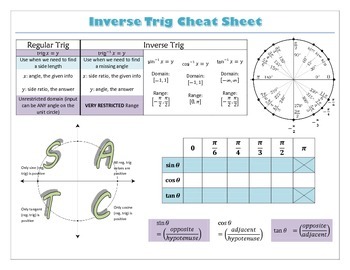
Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,41,N
Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,43,N
Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,45,N
Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,47,N
Reduced Version : http://tutorial.math.lamar.edu/getfile.aspx?file=B,35,N